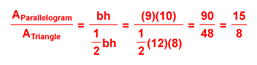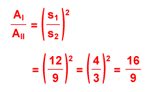|
| you are here > Class Notes - Chapter 11 - Lesson 11-7 | |||||||||||||||||||||||||||||||||||
|
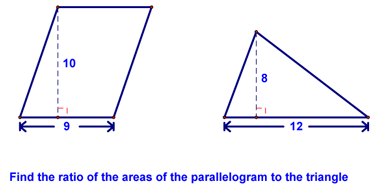
Ratios of Areas - Lesson 11-7 Today we looked at the ratio of areas of figures. We can start with the following example:
Which is pretty straight forward to answer:
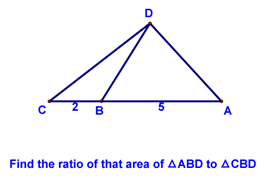
Now look at a second example:
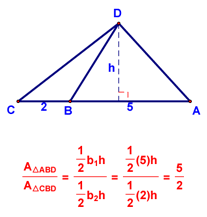
Which is a little less straight forward, but still doable with the realization that both triangles had the same height, which then cancelled out when we did the algebra:
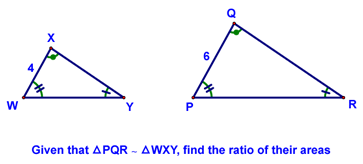
Next, consider the following example:
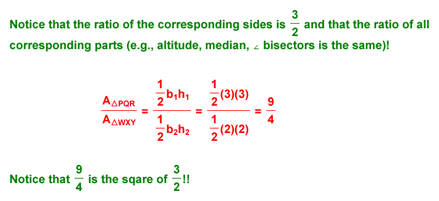
This is interesting...all we have is similar triangles with the ratio of corresponding sides. However, that is enough information to solve the problem!
This fact turns out to hold true for all similar polygons:
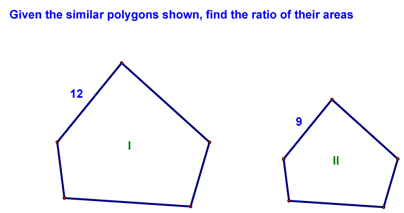
This theorem makes problems of this sort pretty easy. Here's an example:
and its solution!
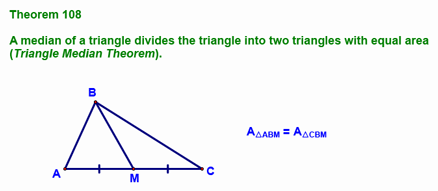
There is one more theorem for today:
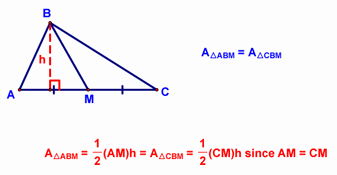
This should make sense to you if you think about it and look at the following:
|
|
||||||||||||||||||||||||||||||||||